After a brief break, we are back to the game theory topic. We know what a dominant strategy is. In a game, it is a player’s go-to strategy, regardless of what the other players do. The most famous example is the Prisoner’s dilemma, where Prisoner 1 has a dominant strategy – to betray—regardless of what Prisoner 2 does. There are two types of dominance.
A strictly dominant strategy: Always provide the player with a greater (never equal to) payoff.

A weakly dominant strategy: Provide at least as good as the other strategies. At least one payoff is strictly greater.
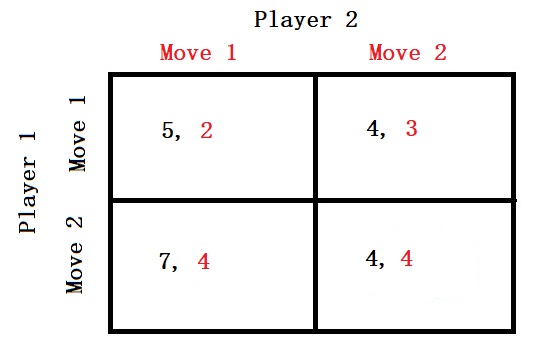
In this game, from Player 1’s perspective, if Player 2 makes move 1, Player 1 gains a better payoff by making move 2 (7 > 5). On the other hand, if Player 2 makes move 2, Player 1 has no strictly better payoff (between move 1 and move 2). So, player 1 is good to make the move 2; however, it is a weakly dominant strategy.

