Ana, Becky and Claire are three singers entering a contest. Ana has won 4% of past competitions, Becky has 5%, and Claire has 6%. If Ana has submitted 7 albums, Becky 2 and Claire 3, what is the probability that Ana will win this time?
The general formula of Bayes’ theorem is:
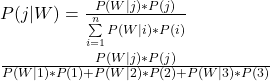
In the present case, for Ana, it is:
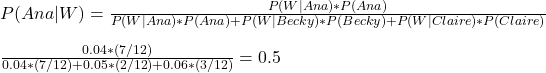
50% chance!

