Let’s go one step further in the expected utility story. Here, we use the same utility function, I1/2, but a different probability of success. This time, the gamble has a 70% chance to get 100,000 vs. 30% chance to lose everything. The expected value is
0.7 x 100,000 + 0.3 x 0 = 70,000
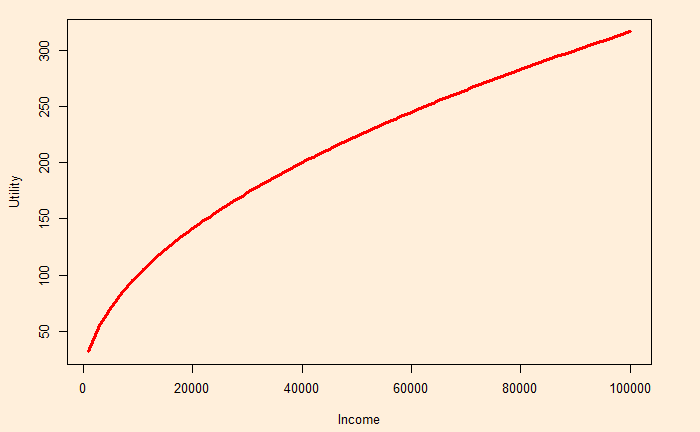
The expected utility is:
0.7 x(100,000)1/2 + 0.3 x 0 = 221.36
Imagine someone guarantees the expected value (70,000). The utility of this amount is:
70,0001/2 = 264.58
Surely, the second person, who is guaranteed the value, is happier. In other words, the risk is removed, or certainty is added in the second case. So, the question is: what is the price of that ‘insurance’?

