Two competing beach vendors offer similar products at similar prices. They can set up their shops wherever they want along the beach. Beachgoers will buy from whichever is closer to them. What is the ideal location for each?
Let the beach be a straight line from 0 to 1, and the shops are somewhere along that line. There are infinite positions for each, and let’s check one of the realisations.

Vendor 1 (V1) gets all businesses on the left side of location V1 and part of the space between V1 and V2. This is marked by the colour red. Vendor 2 (V2) receives all the right and part of the left. The colour green marks this.
Imagine V1 gets into the middle. It acquires at least 50% of the business (the space on the left of V1) and possibly more, depending on where V2 is. Note that V2 can be anywhere on the right of V1.
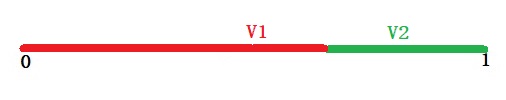
Therefore, V1 is always guaranteed a strategy to get at least half of the business regardless of what V2 does. The same should be true for V2, which can also guarantee half of the business by posting in the centre. Mathematically,
V1 >/= 1/2
V2 >/= 1/2
V1 + V2 = 1
These three equations imply that V1 = V2 = 1/2. This is only possible if they are in the same position or are equidistant from the centre.

If the above is a possibility, then V1 can move towards the right to increase its business. The same is possible if both are in the same location anywhere but in the centre. If they are both located at the centre, they are in Nash equilibrium. If either one moves anywhere else, it will cause some business to lose.

