The foundation of cooperation is not really trust, but the durability of the relationship
Robert Axelrod, The Evolution of Cooperation
We have seen the right strategy for the basic prisoner’s dilemma problem with one play. Defect, and get the better incentive no matter what the other player does. But when it comes to an infinite game, where the same two players play several games, there can be strategies that can make better payoffs than just defect, defect, defect!
University of Michigan Professor of Political Science and Public Policy Robert Axelrod invited experts from game theory to submit programs for a computer infinite prisoner’s dilemma game. Prof Axelrod would then pair off the strategies and find the winner. The winning design was the so-called Tit for Tat, in which the player starts with cooperation and then mirrors what the other player does.
Reciprocation
Let’s see five games between A and B in which A follows the Tit for Tat method. The payoff is similar to what we have seen in the previous post.
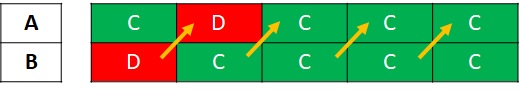
As expected, A starts with cooperation but, seeing B defected, changes to defect in the second game. B realises that and continues cooperating, leading to 13 points for each.
Here is the game with two Tit-for-Tat gamers, both starting with cooperation.
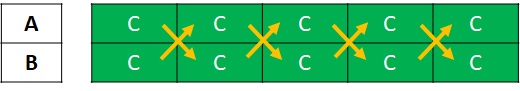
Very peaceful game, and each ends up with 15 points. Imagine the same game, but, for some reason, B starts with a defection.

The payoffs are fine; each defector gets five each time, but the games will follow an alternative pattern.

